【纪念当年的帖子（2008）】高級數學纲要笔记

乙劍真人

注意：

以下是之前零散帖子的微分总结：

remember, you cant memorize the steps, you need to understand the logic behind the theorem..











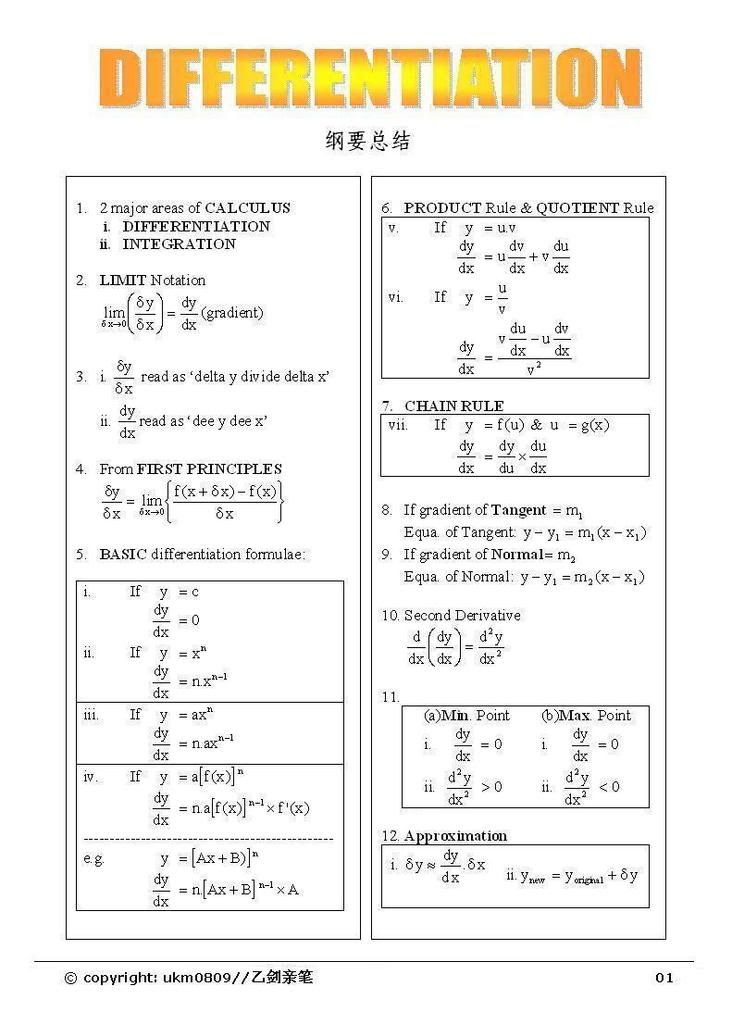
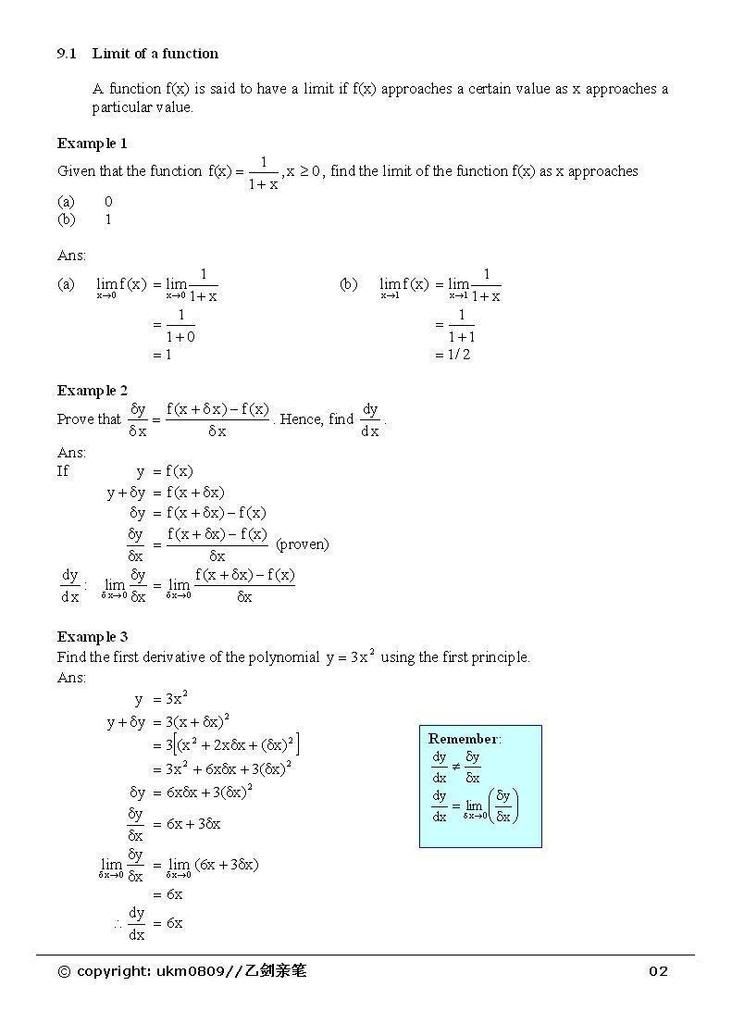
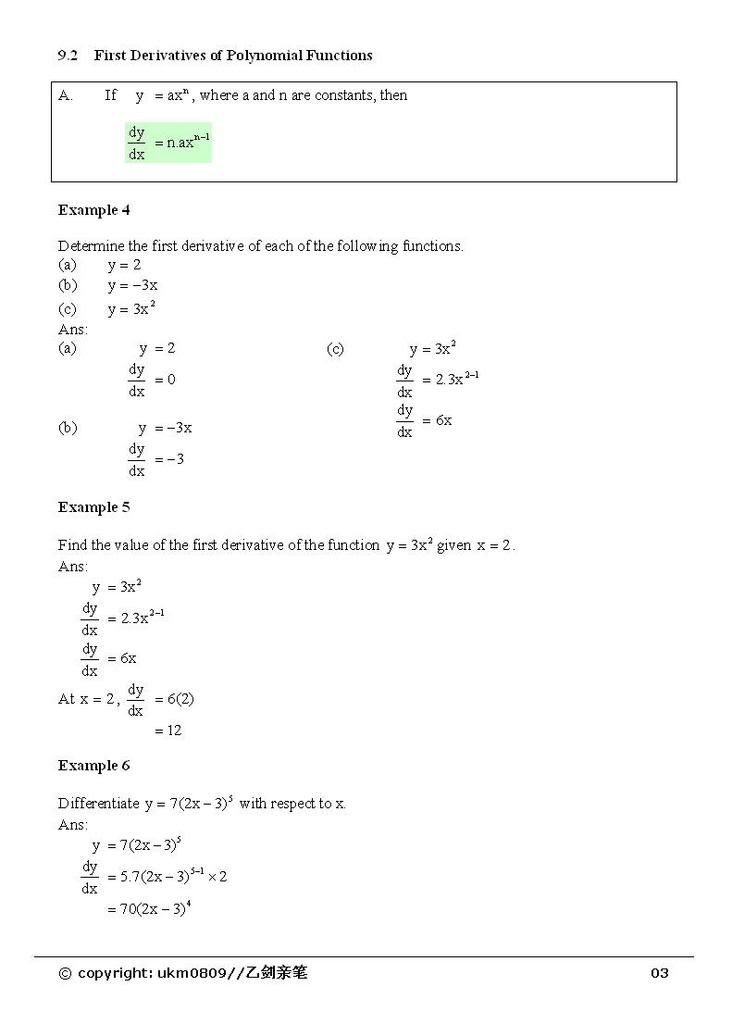
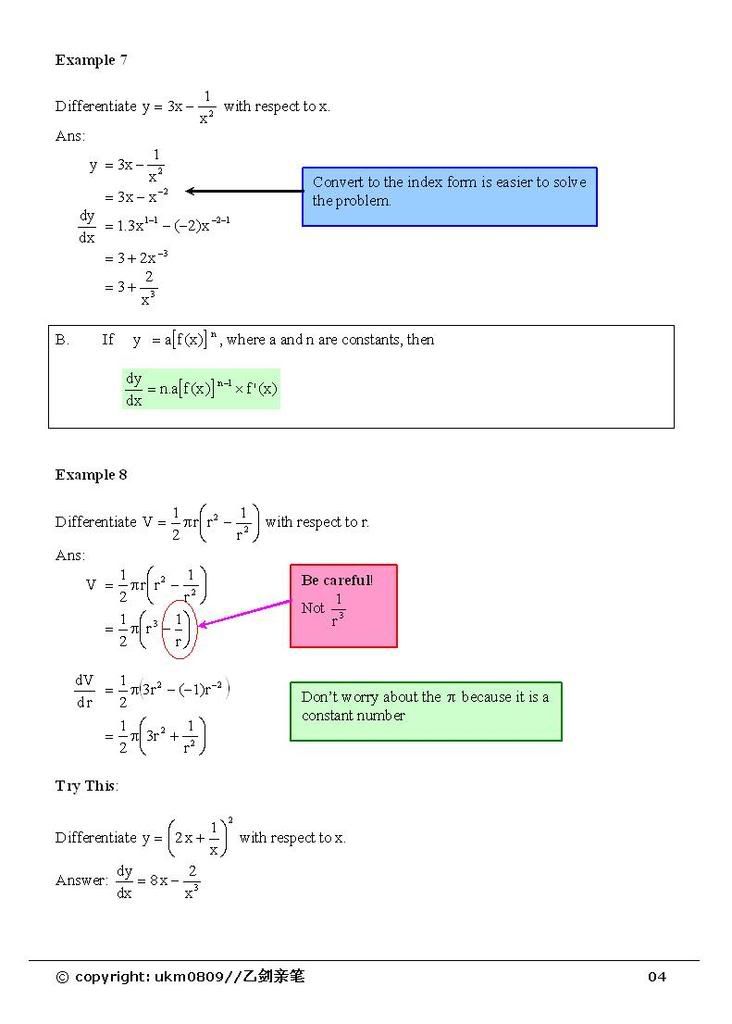
Small Changes and Approximations

我就照白羊的题目讲解这一部分吧..

原帖由 白羊座aries 于 4-10-2008 07:35 PM 发表

Determine the approximate value of square root of 3.99 by using differentiation method

首先：Let y = sqrt x
                   = x power of 1/2
dy/dx = 1/(2sqrt x)

接着，we use x = 4 to substitute into dy/dx because 3.99 approximately to 4.
i.e. dy/dx = 1/4

Delta x = 3.99 - 4 = -0.01

i.e. sqrt 3.99 = sqrt 4 + (dy/dx x Delta x)
                      = 2 + 1/4(-0.01)
                      = 2 - 0.0025
                      = 1.9975

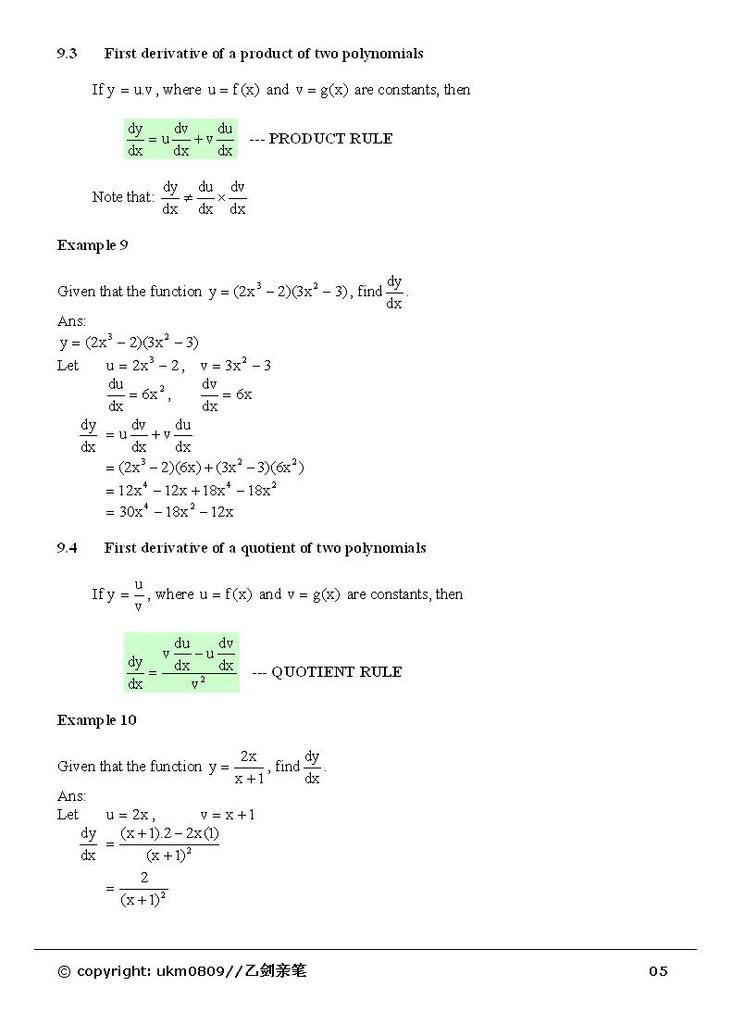
9.5 Equation of the tangent to a curve at a point

Formula：y - y1 = m(x-x1)

Example 11：

Find the equation of the tangent to the curve y = x^2 - 4x + 3 at point (3,4).

Solution：
y = x^2 - 4x + 3
dy/dx = 2x  - 4
At point (3,4), dy/dx = 2(3) - 4 = 2
i.e. gradient of the tangent(m) = 2
therefore, equation of the tangent at point (3,4) is
y - 4 = 2(x - 3)
        = 2x - 6
     y = 2x - 2


9.6 Equation of the normal to a curve at a point

Formula：y - y1 = n(x-x1)

Example 12：

Find the equation of the normal to the curve y = x^2 - 4x + 3 at point (3,4).

Solution：
y = x^2 - 4x + 3
dy/dx = 2x  - 4
At point (3,4), dy/dx = 2(3) - 4 = 2
i.e. gradient of the tangent(m) = 2
      gradient of the normal(n) = -1/2 （m x n = -1)
therefore, equation of the normal at point (3,4) is
y - 4 = -1/2(x - 3)
        = -x + 3
   2y = -x + 11

9.7 Rates of Change

In cases where three variables are involved, say y = f(x) and x = f(t), then
dy/dt = dy/dx x dx/dt

Example 13：

The radius of a sphere increases at a rate of 0.7 cm s ^-1.
At the instant when its radius is 6 cm, find the rate of change of
(a) the volume of the sphere
(b) the total surface area of the sphere

Solution：
Let r, V and A be the radius, volume and total surface area of the sphere respectively.

(a)          V = 4/3 pi r ^3
        dV/dr = 4 pi r ^2
        dV/dt = dV/dr x dr/dt
                  = 4 pi r ^2 x 0.7
                  = 2.8 pi r ^2
When r = 6 cm, dV/dt = 2.8 pi(6^2)
                                     = 100.8 pi cm ^3 s ^-1

(b)         A = 4 pi r ^2
       dA/dr = 8 pi r
       dA/dt = dA/dr X dr/dt
                 = 8 pi r x 0.7
                 = 5.6 pi r
When r = 6 cm, dA/dt = 5.6 pi(6)
                                     = 33.6 cm ^2 s ^-1

9.8 Second Derivative

d/dx(dy/dx) = d^2y/dx^2



Example 14：

Find the turning points for the curve with equation y = x ^3 + x ^2 - x + 5.
Hence, determine the type of turning points.

Solution：
y = x ^3 + x ^2 - x + 5
dy/dx = 3x ^2 + 2x - 1

For turning points, dy/dx = 0
x = -1, x = 1/3

Substitute x = -1 and x = 1/3 into equation y respectively.
i.e. when x = -1, y = 6
      when x = 1/3, y = 130/27

The turning point are (-1,6) and (1/3, 130/27)

d^2y/dx^2 = 6x + 2

For (-1, 6), d^2y/dx^2 = -4 <0
i.e. (-1, 6) is maximum point

For (1/3, 130/27), d^2y/dx^2 = 4 >0
i.e. (1/3, 130/27) is minimum point

以上仅属于一般复习的题目，欲更了解细节，请参考坊间的参考书。





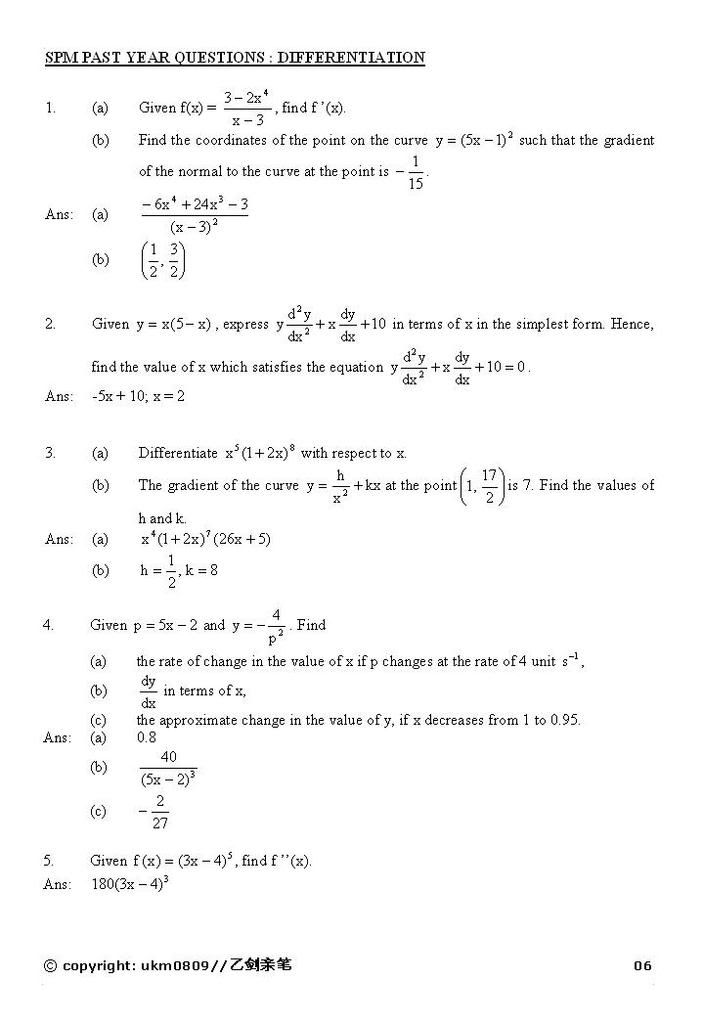
以上乃微分很小的一部分，迟些我再补充资料.. 大家加油！

wkn1ght93

我今年form 4但是我已经学了addmaths两个chapter
有几个问题我不太明白所以希望你们帮我解答

1) The quadratic equation (x+m)^2 = kx has the roots 1 and 16. Find the values of m and the corresponding values of k

2) The roots of quadratic equation 3x^2 + px + 2 = 0 are in the ratio 2:3
   (A) find the value of p if p>0
   (B) hence,find the roots of the equation

3) One of the roots of the equation 9x^2 - 9(k+2)x + 9k^2 + 18k + 11 = 0 is two times the other root. Find the values of k.

乙劍真人

原帖由 wkn1ght93 于 3-1-2009 09:21 PM 发表
我今年form 4但是我已经学了addmaths两个chapter
有几个问题我不太明白所以希望你们帮我解答

(1) The quadratic equation (x+m)^2 = kx has the roots 1 and 16.
      Find the values of m and the corresponding values of k.

SOLUTION:

          Let the roots x = 1 and x = 16, hence substitute the roots into the equation.

          (1 + m)^2 = k(1)
          1 + 2m + m^2 = k                           ---(1)
          (16 + m)^2 = k(16)
          256 + 32m + m^2 - 16k = 0          ---(2)

Substitute (1) into (2):           
          256 + 32m + m^2 - 16(1 + 2m + m^2) = 0
          256 + 32m + m^2 - 16 - 32m - 16m^2 = 0
                                                  -15m^2 + 240 = 0
                                                                       m = +/- 4
When m = 4, k = 1 + 2(4) + (4)^2
                          = 25

When m = - 4, k = 1 + 2(- 4) + (- 4)^2
                            = 9

Hence, the solutions are m = 4, k = 25 ; m = - 4, k = 9

(2) The roots of quadratic equation 3x^2 + px + 2 = 0 are in the ratio 2:3,
     (a) Find the value of p if p>0.
     (b) Hence, find the roots of the equation.

SOLUTION:

(a)     Let the roots x = 2A and x = 3A . Hence, substitute the roots into the equation.
          3(2A)^2 + p(2A) + 2 = 0
          12A^2 + 2Ap + 2 = 0                              ---(1)
          3(3A)^2 + p(3A) + 2 = 0
          27A^2 + 3Ap + 2 = 0                              ---(2)

From (1), p = -6A - 1/A                                     ---(3)

Substitute (3) into (2):
          27A^2 + 3A(-6A - 1/A) + 2 = 0            ---(4)
                  27A^2 - 18A^2 - 3 + 2 = 0
                                               9A^2 = 1
                                                     A = +/- 1/3    ---(5)

When A = 1/3, from (3), p = -6(1/3) - 1/(1/3)
                                         p = -5

When A = -1/3, from (3), p = -6(-1/3) - 1(-1/3)
                                          p = 5
Since p > 0, then p = 5

(b)     Substitute p = 5 into the given equation,
          3x^2 + px + 2 = 0
          3x^2 + 5x + 2 = 0
         (3x + 2)(x + 1) = 0
                               x = -2/3 or x = -1
OR:
          x = 2(-1/3)
             = -2/3

          x = 3(-1/3)
             = -1

(3) One of the roots of the equation 9x^2 - 9(k+2)x + 9k^2 + 18k + 11 = 0 is two times the other root.
      Find the values of k.

SOLUTION:
          9x^2 - 9(k + 2)x + 9k^2 + 18k + 11 = 0

We notice that a = 9, b = -9(k + 2), c = 9k^2 + 18k + 11

Sum Of Roots, SOR = -b/a
                                    = -(-9(k + 2) /9)
                                    = -(-k - 2)
                                    = k + 2
Product Of Roots, POR = c/a
                                         = (9k^2 + 18k + 11)/9

Let the roots x = A and x = 2A because one of the root twice the other one.

SOR, A + 2A = k + 2
                  3A = k + 2
                    A = (k + 2)/3                                        ---(1)

POR,   A(2A) = (9k^2 + 18k + 11)/9                  
               2A^2 = (9k^2 + 18k + 11)/9                    ---(2)

Substitute (1) into (2):
                  2((k + 2)/3)^2 = (9k^2 + 18k + 11)/9
          (2/9)(k^2 + 4k + 4) = (9k^2 + 18k + 11)/9
                  2k^2 + 8k + 8 = 9k^2 + 18k + 11
                7k^2 + 10k + 3 = 0
                 (7k + 3)(k + 1) = 0
                                       k = -3/7 or k = -1

owodog

这贴太棒了！楼主太好了！我支持，大家一起加油

calabash_heng

我高数不及格啊 ！！！！！！！
得温习多点！！！
谢谢楼主的分享

乙劍真人

原帖由 owodog 于 6-1-2009 10:44 PM 发表
这贴太棒了！楼主太好了！我支持，大家一起加油

谢谢你的支持..加油

原帖由 calabash_heng 于 6-1-2009 10:49 PM 发表
我高数不及格啊 ！！！！！！！
得温习多点！！！
谢谢楼主的分享

那你必须多多练习啦..哈哈

希望这里的笔记能够帮上忙..加油！

genesistj

请问这题 if the first two terms of an A.P ARE 1.3 AND 1.9 respestively,find the first term which is greater than 20.7怎样做？ 帮帮忙

乙劍真人

原帖由 genesistj 于 10-1-2009 08:44 PM 发表
请问这题 if the first two terms of an A.P ARE 1.3 AND 1.9 respestively,
find the first term which is greater than 20.7怎样做？ 帮帮忙

嗯..不要哭乖

AP: 1.3, 1.9, ...

a = 1.3, d = 1.9 - 1.3
                 = 0.6

a + (n - 1) d > 20.7
1.3 + (n - 1)0.6 > 20.7
1.3 + 0.6n - 0.6 > 20.7
0.6n > 20
n > 33.33
i.e. n = 34

T34 = 1.3 + (34 - 1) 0.6
        = 21.1

i.e. the first term which is greater than 20.7 is 21.1..

还有一个方法，不过长了点

就是单纯一个加一个，加到号码大过 20.7..

e.g. 1.3, 1.9, 2.5, ...... , 19.9, 20.5, 21.1

genesistj

谢谢 ，原来是这样，我真笨 以后有问题可以再问你吗？？

乙劍真人

原帖由 genesistj 于 11-1-2009 12:16 PM 发表
谢谢 ，原来是这样，我真笨 以后有问题可以再问你吗？？

不客气..

可能你是新人还不习惯类似的问题罢了，只要多练习相信一切问题都能够迎刃而解！加油

如果有问题就放上来吧！

（这里很多高手的哦：hamilan911, vincent5081, ~HeBe~_@ 等都是很有经验又乐意助人的前辈高手）

乙劍真人

Announcement：

大家只需按首页目录就可以浏览每个chapter 的资料！

joyceliew1303

ls的真好……
可见的你是add maths高手
以后不明白什么可以来这里问了……
不知道数学可以吗

乙劍真人

原帖由 joyceliew1303 于 11-1-2009 12:56 PM 发表
ls的真好……
可见的你是add maths高手
以后不明白什么可以来这里问了……
不知道数学可以吗

hamilan911, vincent5081, ~HeBe~_@ 才是真正的好手呢！

他们几位的修为都在我之上呢！

还有在数学论坛的退隐前辈如：微中子，多普勒效应，灰羊，pipi，dunwan2tellu，mathlim，...等等

他们的修为都非同小可，有机会不妨向他们讨教！

happyboy888

好料...太感动了...

Ivanlsy

其他人我不知道，不過mathlim還沒有退隱啦~
他是在潛水~

genesistj

请问下evaluate the geometric series 2+6+...+4374 怎么做？

Ivanlsy

原帖由 genesistj 于 18-1-2009 10:51 AM 发表
请问下evaluate the geometric series 2+6+...+4374 怎么做？

Evaluate the G.S. 2 + 6 + ... + 4374.

First term, a = 2
Common ratio, r = 6/2 = 3

Let nth term, T_n = 4374
              ar^(n - 1) = 4374
           (2)3^(n - 1) = 4374
                3^(n - 1) = 2187
                3^(n - 1) = 3^7
                      n - 1 = 7
                            n = 8

2 + 6 + ... + 4374 = {2[(3^8) - 1]} / 3 -1
                              = (3^8) - 1
                              = 6560

[ 本帖最后由 Ivanlsy 于 18-1-2009 02:11 PM 编辑 ]

genesistj

原来是这样，谢谢你

TKCboy

真行啊！！！
有问必答！！！
好好！！！！！

joyceliew1303

问几题问题……

1.the nth term of a series is 2 to the power of n-1+2n-1.show that the series is not an ap.

2.write down the first 3 terms and find the common difference if the nth term of an ap is
a)3n-7
b)3n/2+12