|
|
 发表于 25-8-2004 02:25 AM
|
显示全部楼层
发表于 25-8-2004 02:25 AM
|
显示全部楼层
好像很静。 丢颗炸弹进来热闹热闹一下。
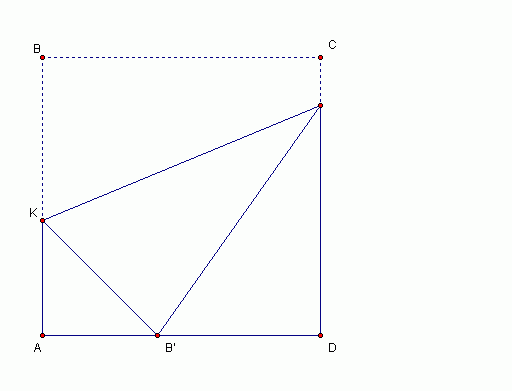
ABCD 是一个正方形的纸。
AB = BC = CD = DA = 10cm
若我把 B 点折下来放在边 AD 上。
找出 AB' 的长度以让面积KAB'是最大。 |
|
|
|
|
|
|
|
|
|
|
|
 发表于 26-8-2004 01:52 AM
|
显示全部楼层
发表于 26-8-2004 01:52 AM
|
显示全部楼层
来解题囖!
1. 设 cos x + sin x =2, (0<x< 90)
求 cos x + sin x = ? |
|
|
|
|
|
|
|
|
|
|
|
 发表于 26-8-2004 01:53 AM
|
显示全部楼层
发表于 26-8-2004 01:53 AM
|
显示全部楼层
山羊座 于 26-8-2004 01:52 说 :
1. 设 cos x + sin x =2, (0<x< 90)
求 cos x + sin x = ?
对不起
打错题目
应为
设 cos x + 3sin x =2, (0<x< 90)
求 cos x + sin x = ? |
|
|
|
|
|
|
|
|
|
|
|
 发表于 26-8-2004 01:55 AM
|
显示全部楼层
发表于 26-8-2004 01:55 AM
|
显示全部楼层
希望解题者可以把过程写出来,
最好在不需应用calculator 的情况下 |
|
|
|
|
|
|
|
|
|
|
|
 发表于 26-8-2004 02:20 PM
|
显示全部楼层
发表于 26-8-2004 02:20 PM
|
显示全部楼层
山羊座 于 26-8-2004 01:53 AM 说 :
设 cos x + 3sin x =2, (0<x< 90)
求 cos x + sin x = ?
我们可设
cos x + sin x = c > 0 (因为 0<x<90 度)
那么 (cos x + sin x)^2 = c^2
所以 (cos x)^2 + (sin x)^2 + 2sin x = c^2
1 + 2 sin x = c^ 2
2 sin x = c^2 - 1
由题目:
cos x + 3sin x =2,
(cos x + sin x) + (2 sin x) = 2
c + c^2 - 1 = 2
(c + 1/2)^2 = 3 + 1/4 = 13/4
c = -1/2 + sqrt(13)/2 or -1/2 - sqrt(13)/2(不可能)
所以 c = {sqrt(13)-1}/2
证毕!! |
|
|
|
|
|
|
|
|
|
|
|
 发表于 26-8-2004 03:36 PM
|
显示全部楼层
发表于 26-8-2004 03:36 PM
|
显示全部楼层
pipi 于 26-8-2004 14:20 说 :
我们可设
cos x + sin x = c > 0 (因为 0<x<90 度)
那么 (cos x + sin x)^2 = c^2
所以 (cos x)^2 + (sin x)^2 + 2sin x = c^2
1 + 2 sin x = c^ 2
2 sin x = c^2 - 1
由题目:
...
Is (cos x + sin x)^2 = (cosx)^2 + (sinx)^2 + 2sinxcosx
= 1 + sin2x ??
So I thought your solution was wrong
And the answer is [4+ (6)^1/2]/5 |
|
|
|
|
|
|
|
|
|
|
|
 发表于 26-8-2004 08:49 PM
|
显示全部楼层
发表于 26-8-2004 08:49 PM
|
显示全部楼层
|
|
|
|
|
|
|
|
|
|
|
 发表于 26-8-2004 11:14 PM
|
显示全部楼层
发表于 26-8-2004 11:14 PM
|
显示全部楼层
一题 polynomial ,请帮帮忙
Given 11x^2- 8xy + 5y^2= 32 ,
x^2+ y^2 = 8 ,
deduce that 7x^2- 8xy + y^2= 0, and
hence , find all the pairs of values of x, y that satisfy the given equation.
请问这题有谁会?我只会 first part , second part 不会 
[ Last edited by 啊蛋 on 26-8-2004 at 11:19 PM ] |
|
|
|
|
|
|
|
|
|
|
|
 发表于 26-8-2004 11:27 PM
|
显示全部楼层
发表于 26-8-2004 11:27 PM
|
显示全部楼层
啊蛋 于 26-8-2004 11:14 PM 说 :
Given 11x^2- 8xy + 5y^2= 32 ,
x^2+ y^2 = 8 ,
deduce that 7x^2- 8xy + y^2= 0, and
hence , find all the pairs of values of x, y that satisfy the given equation.
请问这题有谁会?我只会 ...
第二部分:
7x^2- 8xy + y^2= 0
(7x-y)(x-y)=0
y = x or y = 7x
当 y = x, 由
x^2+ y^2 = 8
x^2+ x^2 = 8
2x^2 = 8
x^2 = 4
x = ±2
当 y = 7x, 由
x^2+ y^2 = 8
x^2+ (7x)^2 = 8
50x^2 = 8
x^2 = 4/25
x = ±2/5
所以,其解为(x,y) = (2,2),(-2,-2),(2/5,14/5),(-2/5,-14/5) |
|
|
|
|
|
|
|
|
|
|
|
 发表于 27-8-2004 12:05 AM
|
显示全部楼层
发表于 27-8-2004 12:05 AM
|
显示全部楼层
|
|
|
|
|
|
|
|
|
|
|
 发表于 28-8-2004 03:09 PM
|
显示全部楼层
发表于 28-8-2004 03:09 PM
|
显示全部楼层
QUESTION :
长方体的一条对角线与各个面所成的角分别为 a,b,c ,
则 (cosa)^2 + (cosb)^2 + (cosc)^2 = _________ . (ANSWER : 2)
Please show the solution for this question! |
|
|
|
|
|
|
|
|
|
|
|
 发表于 29-8-2004 09:38 PM
|
显示全部楼层
发表于 29-8-2004 09:38 PM
|
显示全部楼层
山羊座 于 28-8-2004 03:09 PM 说 :
QUESTION :
长方体的一条对角线与各个面所成的角分别为 a,b,c ,
则 (cosa)^2 + (cosb)^2 + (cosc)^2 = _________ . (ANSWER : 2)
Please show the solution for this question!
我想你的问题应该是"看不到"它要的角度吧!!
试试从以下的图得到一些资料,其他的你自己来吧。。。
应该不难的!!
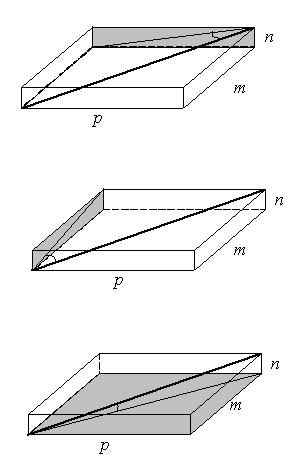
[ Last edited by pipi on 29-8-2004 at 09:39 PM ] |
|
|
|
|
|
|
|
|
|
|
|
 发表于 30-8-2004 07:30 PM
|
显示全部楼层
发表于 30-8-2004 07:30 PM
|
显示全部楼层
persamaan kuadratik ax^2+(a+b)x+b=0 mempunyai dua punca nyata yang berbeza.
(a)Diberi salah satu puncanya ialah 4.Cari punca yang satu lagi.
(b)jika b^2-a^2=3(a-b)dan a 不等于 b ,cari nilai bagi a dan b
Answer:
(a) -1
(b)a= 1, b=-4
谁可以教我做这题(最好有算法和解释) |
|
|
|
|
|
|
|
|
|
|
|
 发表于 31-8-2004 02:44 AM
|
显示全部楼层
发表于 31-8-2004 02:44 AM
|
显示全部楼层
a) katakan punca yg satu lagi= k
maka,4+k=-(a+b)/a dan 4k=b/a
4+k= -1-a/b
=-1-4k
5k=-5
k=-1
b)b^2-a^2=3(a-b)
(b+a)(b-a)=-3(b-a)
b+a=-3 ------------(A)
4k=b/a
4(-1)=b/a
b=-4a --------------(B)
gantikan (B) dlm (A)
-4a+a=-3
-3a=-3
a=1
gantikan a=1 dlm (B)
b=-4(1)
=-4 |
|
|
|
|
|
|
|
|
|
|
|
 发表于 31-8-2004 03:58 PM
|
显示全部楼层
发表于 31-8-2004 03:58 PM
|
显示全部楼层
chwk87 于 31-8-2004 01:44 说 :
a) katakan punca yg satu lagi= k
maka,4+k=-(a+b)/a dan 4k=b/a
4+k= -1-a/b
=-1-4k
5k=-5
k=-1
b)b^2-a^2=3(a-b)
(b+a)(b-a)=-3(b-a)
b+a=-3 ----------- ...
谢谢!!!!! |
|
|
|
|
|
|
|
|
|
|
|
 发表于 31-8-2004 04:22 PM
|
显示全部楼层
发表于 31-8-2004 04:22 PM
|
显示全部楼层
请问这几题如何解答?
1. Diberi persamaan kuadratik (m-3)x^2-(m^2-m-12)x+(3m-1)(m+3)=0. Cari nilai-nilai m jika
(a)salah satu punca bernilai negatif punca yang satu lagi.
(b)salah satu punca merupakan salingan punca yang satu lagi.
2. Cari nilai yang mungkin bagi K jika garis lurus y=7+k ialah tangen kepada lengkungan y=2x^2-3x+2.Seterusnya cari titik di mana sentuhan berlaku.
3. Diberi persamaan kuadratik rx^2-(3p+1)x-(p+5)=0 dan r不等于0.Nyatakan syarat yang harus dipatuhi oleh p supaya persamaan kuadratik ini mempunyai dua punca nyata yang sama.
4. Diberi bahawa A dan B ialah punca-punca bagi persamaan 2x^2=3x-1 dan A>B.
(a)Bentukkan persamaan kuadratik yang mempunyai punca-punca A^4+4B^2+1 dan 16B^2-3A^2.
anwers:
1.(a) m=-3atau4
(b) m=0atau -2(1/3)
2.k=-6(1/8);(3/4,7/8)
3.p不等于-1/3
4.x^2-4x+3=0
对不起,太多题不会做
请各位高手指点!!!!!!
谢谢!!! |
|
|
|
|
|
|
|
|
|
|
|
 发表于 31-8-2004 06:55 PM
|
显示全部楼层
发表于 31-8-2004 06:55 PM
|
显示全部楼层
不好意思,可以请问这题应该怎么解吗?
when the polynomial p(x)is devided by (x-3) the remainder is 3,
and when p(x) is devided by (x+2) the remainder is 13.
Find the remainder when the polynomial is devided by (x-3)(x+2) |
|
|
|
|
|
|
|
|
|
|
|
 发表于 1-9-2004 01:50 AM
|
显示全部楼层
发表于 1-9-2004 01:50 AM
|
显示全部楼层
1 a) katakan satu punca=a,maka punca yg satu lagi=-a
h.t.p = a+(-a) = (m^2-m-12)/(m-3)
0=m^2-m-12
(m-4)(m+3)=0
maka,m=4 @-3
b) katakan satu punca=a ,maka punca yg satu lagi=1/a
h.D.p.=a(1/a) =(3m-1)(m+3)/(m-3)
m-3=3m^2+8m-3
3m^2+7m=0
m(3m+7)=0
maka,m=0 @ -7/3
2)y=7+k -----------1
y=2x^2-3x+2 ----------2
gantikan 1 dlm 2
maka 2x^2-3x-5-k=0
b^2-4ac=0
kamu akn dpt
k=-49/8
kemudian gantikan nilai k dlm (1) dan selesaikan pers serentak itu, kamu akn dpt jwpnya.
3)my own answer is p should be equel to -29/3
may be i did wrong.
4)2x^2-3x+1=0
A+B=3/2
A=3/2-B -----1
AB=-1/2-------2
selesaikan,maka kamu akn dapat B=1/2 @ 1
o krn A>B, maka B=1/2 dan A=1
H.T.P= A^4+4B^2+1+16B^2-3A^2.
=A^4+20B^2+1-3A^2
=1^4+ 20(1/2)^2+1-3(1)^2
=4
H.D.P=(A^4+4B^2+1)(16B^2-3A^2)
=(1^4+4(1/2)^2+1)(16(1/2)^2-3(1)^2)
=3.
maka x^2-(H.T.P)x+(H.D.P)=0
x^2-4x+3=0 |
|
|
|
|
|
|
|
|
|
|
|
 发表于 1-9-2004 09:38 PM
|
显示全部楼层
发表于 1-9-2004 09:38 PM
|
显示全部楼层
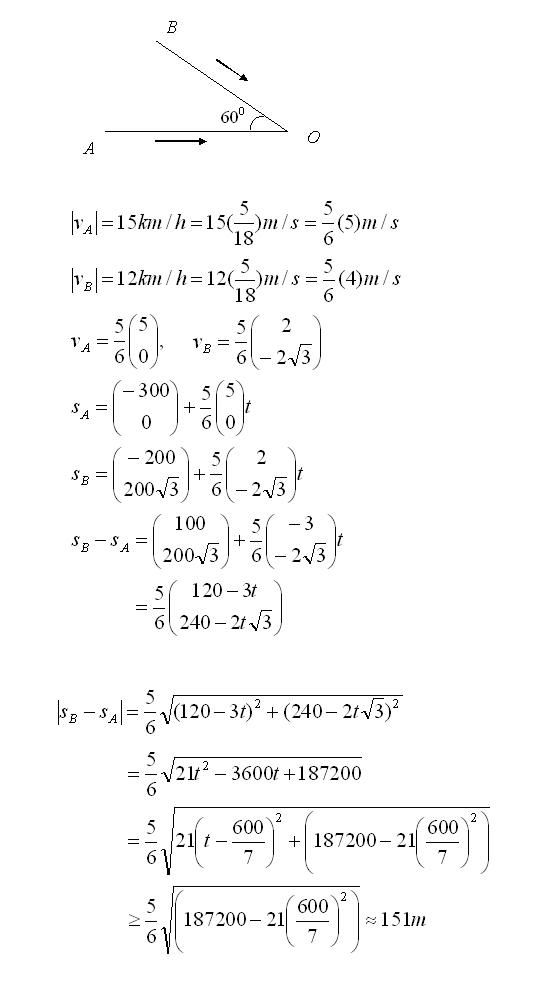
要考trial exam了!请帮忙。。。
Form 6: vector
A lane crosses with another lane at 0 at an angle of 60. A boy, A, is 300m from O in one of the lanes and another boy, B, is 400m from O in another lane. Both the boys run towards O simultaneously, with A moving at a speed of 15km/h and B at a speed of 12km/h. Find the closest distance between A and B.
answer is 151m |
|
|
|
|
|
|
|
|
|
|
|
 发表于 2-9-2004 03:40 PM
|
显示全部楼层
发表于 2-9-2004 03:40 PM
|
显示全部楼层
晴天蓝 于 1-9-2004 09:38 PM 说 :
Form 6: vector
A lane crosses with another lane at 0 at an angle of 60. A boy, A, is 300m from O in one of the lanes and another boy, B, is 400m from O in another lane. Both the boys run towards O ...
我的答案:
 |
|
|
|
|
|
|
|
|
|
| |
 本周最热论坛帖子 本周最热论坛帖子
|